Descriptive Statement

Number sense is defined as an intuitive feel for numbers and a common sense approach to using them. It is a comfort with what numbers represent, coming from investigating their characteristics and using them in diverse situations. It involves an understanding of how different types of numbers, such as fractions and decimals, are related to each other, and how they can best be used to describe a particular situation. Number sense is an attribute of all successful users of mathematics.

Meaning and Importance

Successful users of mathematics have good number sense. When someone chooses to use fractions in one situation and decimals in another because the respective operations are easier to perform or the results are easier to understand, that process is evidence of good number sense. When students continue work on a problem involving numbers until they recognize that their answers are reasonable in the context of the problem, they are using good number sense. When a student is comfortable with using an approximation to a number in certain situations, or understands that an approximation rather than an exact number might have been used, then that reflects good number sense. When students recognize that an answer is off by a factor of ten, or alternately that a decimal point has been misplaced, they are using good number sense.

Our students often do not connect what is happening in their mathematics classrooms with their daily lives. It is essential that the mathematics curriculum build on the sense of number that students bring with them to school. Problems and numbers which arise in the context of the students' world are more meaningful to students than traditional textbook exercises and help them develop their sense of how numbers and operations are used. Frequent use of estimation and mental computation are also important ingredients in the development of number sense, as are regular opportunities for student communication. Discussion of their own invented strategies for problem solutions helps students strengthen their intuitive understanding of numbers and the relationships between numbers.

A "sense-building mode" is best established when students are provided with opportunities to explore number relationships, are encouraged to question and to challenge, and are allowed to experiment to discover strategies and techniques of their own that ease the path to the solution of mathematical problems.

K-12 Development and Emphases

A necessary foundation for a strong number sense is the development of meaning for numbers, beginning with whole numbers, decimals, and fractions. Traditionally, this component of the curriculum has been called numeration, and it is vitally important. The K-12 mathematics curriculum should provide the appropriate experiences, physical models, and manipulatives to assist in the construction of these meanings. Appropriate technology should also be regularly used to help students develop their number sense. Through regular and frequent experiences emphasizing the measurement of real objects, the counting and grouping of sets of discrete objects, and the well designed use of calculators, elementary students develop place value concepts, a sense of magnitude (size), and approximation and estimation skills for whole numbers, decimals, and fractions. Real-world situations should be incorporated into their experiences to help young students become aware of the existence of other numbers, such as negative numbers.

The development of personal meaning for numbers should be reinforced in the middle grades with an extension to other numbers and notations such as integers, percents, exponents, and roots. High school students should extend their meaning of number to the real number system and recognize that still other number systems exist. They should have the opportunity to develop intuitive proofs of the fundamental properties of closure, commutativity, associativity, and distributivity.

Students must develop a facility for working with the different types of numbers we use in day-to-day activities. Statements about one particular quantity might best be expressed with a fraction, a percent, a decimal, a ratio, an approximated whole number, or some other number form; comparisons often have to be made among numbers in different forms. Therefore, students will need to be able to transform numbers from one form to equivalent numbers in another form, and to intelligently select the right form of numbers to use in a particular situation. The correct choice depends on the context of the situation, and therefore students must possess an adequate understanding of each form and the interrelationships among them.

One way to achieve such an understanding in the classroom is through the identification and description of number patterns and the use of pattern-based thinking. For example, examining and modeling many pairs of fractions with equal numerators help students develop the understanding and generalization that the fractions with larger denominators represent smaller quantities. Activities promoting pattern-based thinking can assist students in making similar generalizations about other number forms and their relationships, as well as build initial notions of still other types of important number concepts such as odd and even, prime numbers, and factors and multiples.

Graduates of our schools must be able to use numbers intelligently and understand them wherever they are encountered in real life. They must develop an awareness of numbers and their uses. Numbers are used as counts, measures, labels, and locations, and each use has unique characteristics and restrictions on the appropriate forms and operations. The opportunity to develop the needed familiarity with all of these uses comes through the regular presentation of problem situations which utilize them. Some activities should focus on the explicit uses of the numbers themselves, however. A discussion of why it makes no sense to add the numbers on a town's roadway welcome sign (see diagram on the next page) which lists its population, altitude, and year of founding would serve a dual purpose: to provide an example of some the standard uses of numbers, and to challenge the thoughtless computational manipulation of numbers.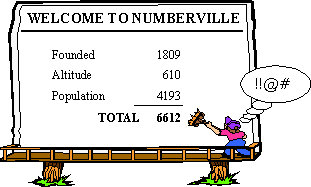

In summary, the commitment to develop number sense requires a dramatic shift in the way students learn mathematics. Our students will only develop strong number sense to the extent that their teachers encourage the understanding of mathematics as opposed to the memorization of rules and mechanical application of algorithms. Every child has the capability to succeed as a user of mathematics, but the degree of success is directly related to the strength of their number sense. The way to assure that all students acquire a good sense of number is to have them consistently engage in activities which require them to think about numbers and number relationships and to make the connection with quantitative information encountered in their daily lives.

Note: Although each content standard is discussed in a separate chapter, it is not the intention that each be treated separately in the classroom. Indeed, as noted in the Introduction to this Framework, an effective curriculum is one that successfully integrates these areas to present students with rich and meaningful cross-strand experiences.