The widespread availability of computing and calculating technology has given us the opportunity to reconceive the role of computation and numerical operations in our third and fourth grade mathematics programs. Traditionally, tremendous amounts of time were spent at these levels helping children to develop proficiency and accuracy with paper-and-pencil procedures. Now, adults needing to perform calculations quickly and accurately have electronic tools that are both more accurate and more efficient than those procedures. At the same time, though, the new technology has presented us with a situation where some numerical operations, skills, and concepts are much more important than they used to be. As described in the K-12 Overview, estimation, mental computation, and understanding the meanings of the standard arithmetic operations all play a more significant role than ever in the everyday life of a mathematically literate adult.

The major shift in the curriculum that will take place in this realm, therefore, is one away from drill and practice of paper-and-pencil procedures with symbols and toward real-world applications of operations, wise choices of appropriate computational strategies, and integration of the numerical operations with other components of the mathematics curriculum.

Third and fourth graders are primarily concerned with cementing their understanding of addition and subtraction and developing new meanings for multiplication and division. They should be in an environment where they can do so by modeling and otherwise representing a variety of real-world situations in which these operations are appropriately used. It is important that the variety of situations to which they are exposed include all the different scenarios in which multiplication and division are used. There are several slightly different taxonomies of these types of problems, but minimally students at this level should be exposed to repeated addition and subtraction, array, area, and expansion problems. Students need to recognize and model each of these problem types for both multiplication and division.

Basic facts in multiplication and division continue to be very important. Students should be able to quickly and easily recall quotients and products of one-digit numbers. The most effective approach to enabling them to acquire this ability has been shown to be the focused and explicit use of basic fact strategies-conceptual techniques that make use of the child's understanding of the operations and number relationships to help recover the appropriate product or quotient. Doubles and near doubles are useful strategies, as are discussions and understandings regarding the regularity in the nines multiplication facts, the roles of one and zero in these operations, and the roles of commutativity and distributivity.

Students must still be able to perform two-digit multiplication and division with paper and pencil, but the widespread availability of calculators has made the particular procedure used to perform the calculations less important. It need no longer be the single fastest, most efficient algorithm chosen without respect to the degree to which children understand it. Rather, the teaching of two-digit computation should take on more of a problem solving approach, a more conceptual, developmental approach. Students should first use the models of multi-digit numbers that they are most comfortable with (base ten blocks, money) to explore this new class of problems. Students who have never formally done two-digit multiplication might be asked to use their materials to help figure out how many pencils are packed in the case just received in the school office. There are 24 boxes with a dozen pencils in each box. Are there enough for everystudent in the school to have one? Other, similar, real-world problems would follow, some involving regrouping and others not.

After initial exploration, students share with each other all of the strategies they've developed, the best ways they've found for working with the tens and ones in the problem, and their own approaches to dealing with the place value issues involved. Most students can, with direction, take the results of those discussions and create their own paper-and-pencil procedures for multiplication and division. The discussions can, of course, include the traditional approaches, but these ought not to be seen as the only right way to perform these operations.

Estimation and mental math become critically important in these grade levels as students are inclined to use calculators for more and more of their work. In order to use that technology effectively, third and fourth graders must be able to use estimation to know the range in which the answer to a given problem should lie before doing any calculation. They also must be able to assess the reasonableness of the results of a computation and be satisfied with the results of an estimation when an exact answer is unnecessary. Mental mathematics skills, too, play a more important role in third and fourth grade. Simple two-digit addition and subtraction problems and those involving powers of ten should be performed mentally. Students should have enough confidence in their ability with these types of computations to do them mentally instead of relying on either a calculator or paper and pencil.

Technology should be an important part of the environment in third and fourth grade classrooms. Calculators provide a valuable teaching tool when used to do student-programmed repeated addition or subtraction, to offer estimation and mental math practice with target games, and to explore operations and number types that the students have not yet formally encountered. Students should also use calculators routinely to find answers to problems that they might not be able to find otherwise. This use prevents the need to artificially contrive real-world problems so that their answers are numbers with which the students are already comfortable.

The topics that should comprise the numerical operations focus of the third and fourth grade mathematics program are:

multiplication and division basic facts

multi-digit whole number addition and subtraction

two-digit whole number multiplication and division

decimal addition and subtraction

explorations with fraction operations

The cumulative progress indicators for grade 4 appear below in boldface type. Each indicator is followed by activities which illustrate how it can be addressed in the classroom in grades 3 and 4.

Building upon knowledge and skills gained in the preceding grades, experiences in grades 3-4 will be such that all students:

1. Develop meaning for the four basic arithmetic operations by modeling and discussing a variety of problems.

• Students broaden their initial understanding of multiplication as repeated addition by dealing with situations involving arrays, expansions, and combinations. Questions of these types are not easily explained through repeated addition: How many stamps are on this 7 by 8 sheet? How big would this painting be if it was 3 times as big? How many outfits can you make with 2 pairs of pants and 3 shirts?

• Students use counters to model both repeated subtraction (There are 12 cookies. How many bags of 3?) and sharing (There are 12 cookies and 3 friends. How many cookies each?) meanings for division and write about the difference in their journals.

• Students work through the Sharing Cookies lesson that is described in the First Four Standards of this Framework. They investigate division by using 8 cookies to be shared equally among 5 people, and discuss the problem of simplifying the number sentence which describes the amount of each person's share.

• From the beginning of their work with division, children are asked to make sense out of remainders in problem situations. The answers to these three problems are different even though the division is the same: How many cars will we need to transport 19 people if each car holds 5? How many more packages of 5 ping-pong balls can be made if there are 19 balls left in the bin? How much does each of 5 children have to contribute to the cost of a $19 gift?

• Students explore division by reading The Doorbell Rang by Pat Hutchins. In this story, Victoria and Sam must share 12 cookies with increasing numbers of friends. Students can use counters to show how many cookies each person gets.

• Students learn about multiplication as an array by reading One Hundred Hungry Ants by Elinor Pinczes, Lucy and Tom's 1, 2, 3 by Shirley Hughes or Number Families by Jane Srivastava.

• Students make books showing things that come in 3's, 4's, 5's, 6's, or 12's.

2. Develop proficiency with and memorize basic number facts using a variety of fact strategies (such as "counting on" and "doubles").

• Students use streets and alleys as both a mental model of multiplication and a useful way to recover facts when needed. It simply involves drawing a series of horizontal lines(streets) to represent one factor and a series of vertical lines (alleys) crossing them to represent the other. The number of intersections of the streets and alleys is the product!

• Students use a double maker on a calculator for practice with doubles. They enter x 2 = on the calculator. Any number pressed then, followed by the equal sign, will show the number's double. Students work together to try to say the double for each number before the calculator shows it.

• Students regularly use doubles, near doubles, and use a related fact strategies for multiplication; they are using the near doubles strategy when they calculate a sum like 15 + 17 by recognizing that it is 2 more than double 15. More generally, they are using the use a related fact strategy when they use any fact they happen to remember, like 8 + 4 = 12, to make a related calculation like 8 + 5 =12 + 1 = 13. They also recover facts by knowledge of the role of zero and one in multiplication, of commutativity, and of the regular patterned behavior of multiples of nines. Practice sets of problems are structured so that use of all these strategies is encouraged and the students are regularly asked to explain the procedures they are using.

• Pairs of students play Circles and Stars (Burns, 1991). Each student rolls a die and draws as many circles as the number shown, then rolls again and puts that number of stars in every circle, and then writes a multiplication number sentence and records how many stars there are all together. Each student takes seven turns, and adds the total. The winner is the student with the most stars.

• Students use color tiles to show how a given number of candies can be arranged in a rectangular box.

• Students play multiplication war, using a deck of cards with kings and queens removed. All of the cards are dealt out. Each player turns up two cards and multiplies their values (Jacks count as 0; aces count as 1). The "general" draws a target number from a hat. The player closest to the target wins a point. The first player to get 10 points wins the game.

• Students use computer programs such as Math Workshop to practice multiplication facts.

3. Construct, use, and explain procedures for performing whole number calculations in the various methods of computation.

• Students work through the Product and Process lesson that is described in the Introduction to this Framework. It challenges students to use calculators and four of the five digits 1, 3, 5, 7, and 9 to discover the multiplication problem that gives the largest product.

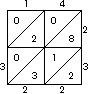• Students explore lattice multiplication and try to figure out how it works. For example, the figure at the right shows 14 × 23 = 322.

• Students use the skills they've developed with arrow puzzles (See Standard 6-Number Sense-Grades 3-4-Indicator 3) to practice mental addition and subtraction of 2- and 3-digit numbers. To add 23 to 65, for instance, they start at 65 on their "mental hundred number chart," go down twice and to the right three times.

• Students use base ten blocks to help them decide how many blocks there would be in eachgroup if they divided 123 blocks among 3 people. The students describe how they used the blocks to help them solve the problem and compare their solutions and solution strategies.

4. Use models to explore operations with fractions and decimals.

• Students use fraction circle pieces (each unit fraction a different color) to begin to explore addition of fractions. Questions like: Which of these sums are greater than 1? and How do you know? are frequent.

• Students use the base ten models that they are most familiar with for whole numbers and relabel the components with decimal values. Base ten blocks represent 1 whole, 1 tenth, 1 hundredth, and 1 thousandth. Coins, which had represented a whole number of cents, now represent hundredths of dollars.

• Students operate a school store with school supplies available for sale. Other students, using play money, decide on purchases, pay for them, receive and check on the amount of change.

• In groups, students each roll a number cube and use dimes to represent the decimal rolled. For example, a student rolling a 4 would take 4 dimes to represent 4 tenths of a dollar. When a student gets 10 dimes, he turns them in for a dollar. The first student to get $5 wins the game.

• Students use money to represent fractions. For example, a quarter and a quarter equals half a dollar.

• Students demonstrate equivalent fractions using pattern blocks. For example, if a yellow hexagon is one whole, then three green triangles (3/6) is the same size as one red trapezoid (1/2). Pattern blocks may also be used to represent addition and subtraction of fractions.

5. Use a variety of mental computation and estimation techniques.

• Students frequently do warm-up drills that enhance their mental math skills. Problems like: 3,000 x 7 = , 200 x 6 = , and 5,000 x 5 + 5 = are put on the board as individual children write the answers without doing any paper-and-pencil computation.

• Students make appropriate choices from among front-end, rounding, and compatible numbers strategies in their estimation work depending on the real-world situation and the numbers involved. Front end strategies involve using the first digits of the largest numbers to get an estimate, which of course is too low, and then adjusting up. Compatible numbers involves finding some numbers which can be combined mentally, so that, for example, 762 + 2,444 + 248 is about (750 + 250) + 2,500, or 3,500.

• Students use money and shopping situations to practice estimation and mental math skills. Is $20.00 enough to buy items priced at $12.97, $4.95, and 3.95? About how much would 4 cans of beans cost if each costs $0.79?

• Students explore estimation involving division as they read The Greatest Guessing Game: A Book about Dividing by Robert Froman. A little girl and her three friends solve a variety of problems, estimating first and discussing what to do with remainders.

6. Select and use appropriate computational methods from mental math, estimation, paper-and-pencil, and calculator methods, and check the reasonableness of results.

• Students play addition max out. Each student has a 2 x 3 array of blanks (in standard 3-digit addition form) into each of which will be written a digit. One student rolls a die and everyone must write the number showing into one of their blanks. Once the number is written in, it can not be changed. Another roll - another number written, and so on. The object is to be the player with the largest sum when all six digits have been written. If a player has the largest possible sum that can be made from the six digits rolled, there is a bonus for maxing out.

• Students discuss this problem from the NCTM Standards (p. 45): Three fourth grade teachers decided to take their classes on a picnic. Mr. Clark spent $26.94 for refreshments. He used his calculator to see how much the other two teachers should pay him so that all three could share the cost equally. He figured they each owed him $13.47. Is his answer reasonable? As a follow-up individual assessment, they write about how they might find an answer.

7. Understand and use relationships among operations and properties of operations.

• Students take 7x8 block rectangular grids printed on pieces of paper. They each cut along any one of the 7 block-long segments to produce two new rectangles, for example, a 7x6 and a 7x2 rectangle. They then discuss all of the different rectangle pairs they produced and how they are all related to the original one.

• Students write a letter to a second grader explaining why 2+5 equals 5+2 to demonstrate their understanding of commutativity.

• Students explore modular, or clock, addition as an operation that behaves differently from the addition they know how to do. For example: 6 hours after 10 o'clock in the morning is 4 o'clock in the afternoon, so 10 + 6 = 4 on a 12-hour clock. How is clock addition different from regular addition? How is it the same? How would modular subtraction and multiplication work?

References

Burns, Marilyn. Math By All Means: Multiplication, Grade 3. New Rochelle, New York: Cuisenaire, 1991.

Froman, Robert. The Greatest Guessing Game: A Book About Dividing. New York: Thomas Y. Crowell Publishers, 1978.

Hughes, Shirley. Lucy and Tom's 1, 2, 3. New York: Viking Kestrel, 1987.

Hutchins, Pat. The Doorbell Rang. New York: Greenwillow Books, 1986.

National Council of Teachers of Mathematics. Curriculum and Evaluation Standards for School Mathematics. Reston, VA, 1989.

Pinczes, Elinor J. One Hundred Hungry Ants. Boston: Houghton Mifflin Company, 1993.

Srivastava, Jane. Number Families. New York: Thomas Y. Crowell, 1979.

Software

Math Workshop. Broderbund.

On-Line Resources

http://dimacs.rutgers.edu/archive/nj_math_coalition/framework.html/

The Framework will be available at this site during Spring 1997. In time, we hope to post additional resources relating to this standard, such as grade-specific activities submitted by New Jersey teachers, and to provide a forum to discuss the Mathematics Standards.