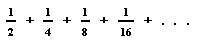Seventh and eighth graders should have a good sense of whole numbers and their orders of magnitude and should be focusing on further developing number sense with decimals and fractions. They should be extending their understanding of whole numbers to negative numbers, including comparison and ordering. They also should be working on incorporating ratio, proportion and percent, powers and roots, and scientific notation into their conception of the number system. The key components of number sense, as explained in the K-12 Overview, are an awareness of the uses of numbers in the world around us, a good sense of approximation, estimation, and magnitude, the concept of numeration, and an understanding of comparisons and the equivalence of different representations and forms of numbers.

Students at this age are capable of categorizing the ways in which numbers are used in our society. One interesting activity is to have them collect data on how numbers appear in a portion of a newspaper, categorize these uses, and then graph their results.

In their work with numeration, seventh- and eighth-graders should begin to see mathematics as a coherent body of knowledge. They should begin to see the integers and the rationals as logical and necessary extensions of the whole number system. Only with these extensions can expressions like 3 -7 and 4 divided by 3 have answers.

In grades 7 and 8, students are focusing on ratios, proportions, and percents, topics which they were just beginning to consider in grades 5 and 6. Their work with these concepts and the relationship of these numbers to fractions, decimals, and whole numbers form the foundation for a very powerful problem solving skill: proportional reasoning. New topics at these grade levels are exponents, roots, and scientific notation. Students should also explore irrational numbers, such as pi, square roots of numbers which are not perfect squares, and other decimals which neither end nor repeat.

Students at these grade levels need to continue learning about equivalence, but there is a vast array of kinds of equivalence to be considered here. Students focus on the representation of the same quantity using different types of numbers and the selection of the appropriate number type given a particular problem context. It is particularly important that students understand the difference between the exact value of a fraction, such as 2/3, and its approximation of .667, especially since they now use calculators routinely. Relationships among decimals, fractions, ratios, and percents comprise the largest emphasis, but work with exponents and roots and their relationship to scientific notation is also a focus in these grades. Number theory provides a rich context for interesting problems in this area. Questions about infinity, division by zero, and primes and composites, combine with discussions about finite and infinite sequences and series and searches for patterns to open up the full richness of a mathematical world.

Estimation should be a routine part of mathematics classes. Students should be regularly engaged in estimating both quantities and the results of operations. They should respond to questions that arise naturally during the course of the class with answers which demonstrate confident and well-conceived use of estimation strategies and sense of number.

Technology plays an important role in number sense at these grade levels. Calculators can be wonderful exploration tools when examining numerical relationships. Many insights about the relationships between fractions and decimals, for instance, can be attained by simply dividing the numerators of fractions by their denominators. Generalizations about what kinds of fractions produce what kinds of decimals start to flow very freely in such open-ended explorations. Computer software can also be very useful. Spreadsheets, for example, can show a great many ratios on the screen at the same time. For example, the five ingredients of a waffle recipe that makes 4 waffles can be listed across the top row of the spreadsheet, with following rows showing how the recipe changes to make 2, 8, and 12 waffles (Curriculum and Evaluation Standards, 1989, p. 89).

The topics that should comprise the number sense focus of the seventh and eighth grade mathematics program are:

rational numbers (both positive and negative)

equivalence

integers

ratio, proportion, and percent

exponents, roots, and scientific notation

The cumulative progress indicators for grade 8 appear below in boldface type. Each indicator is followed by activities which illustrate how it can be addressed in the classroom in grades 7 and 8.

Building upon knowledge and skills gained in the preceding grades, experiences in grades 7-8 will be such that all students:

10. Understand money notations, count and compute money, and recognize the decimal nature of United States currency.

• Students can solve a variety of real-world money problems such as: If you make $750.00 a month, would you rather have a 12% raise or an $85 a month raise? or Which sale is better on a $17.00 sweater, a 1/3 off sale, a $5.00 discount, or a 30% discount?

• Students use time-cards and pay rates to compute weekly wages and deductions for various workers. Issues such as, time-and-a-half for overtime, double time for holidays, and percentages of total wages to be deducted for various taxes all come into play.

• Students investigate the details and make plans to dispose of the proceeds of the $27,000,000 lottery which they just won.

11. Extend their understanding of the number system by constructing meanings for integers, rational numbers, percents, exponents, roots, absolute values, and numbers represented in scientific notation.

• Students develop a scientific notation Olympics by creating events like the 9.144 x 10³ centimeter sprint (100 yard dash) or the 7.272 x 10⁶ milligram hurl (shot put).

• Students use a bookkeeping simulation to explore the effect of bills and credits coming into, or going out of, their business. These financial activities are recorded as various actions on positive and negative integers, all affecting the net worth of the business.

• Students view the Powers of Ten video, developed to show how one's view of the world is affected by changes in the order of magnitude of one's position.

• Students construct a hypothetical stock portfolio, using $10,000 to "buy" shares of stock, and track the performance of the portfolio and each individual stock day by day.

• Students explore absolute values as the distance between two points on a number line and compare this to subtraction.

• Students measure the circumference and diameter of 15 to 20 round objects, recording the results in a table. They make a scatterplot (with diameter on the horizontal axis) and use a piece of spaghetti to draw the line of best fit. They discuss why pi is represented in the drawing as the slope of the line.

• Students construct line segments of varying irrational lengths on a geoboard or dot paper. For example, the diagonal of a unit square has length sqrt(2), the diagonal of a 1 x 2 rectangle has length sqrt(5), and the circumference of a circle with unit diameter has length pi. Through exploring these common irrational numbers that arise in problem situations, students learn that not all numbers can be represented as a ratio of two integers.

• Students construct their own graph for the square roots of the numbers from 1 to 25, using trial-and-error to approximate each root to the nearest tenth. They plot the numbers on the horizontal scale, and their square roots on the vertical scale.

• Students work on traditional "systems of equations" problems, involving two unknowns, by devising non-algebraic solution strategies for them. Some samples are: Two numbers have a sum of 32; they have a product of 240. What are the numbers? or Sally is 22 years younger than her Dad. In 3 years, her Dad will be 3 times as old as she. How old is Sally?

12. Develop number sense necessary for estimation.

• Students wrestle with this classic problem: After spending most of the day looking for her missing pet cat, Whiskers, the eccentric billionaire, Ms. Money Bags, received a ransom demand. The caller said she was to bring a suitcase packed with $1,000,000 in one- and five-dollar bills to the bus station and leave it in Locker #26-C. Then her pet would be returned to her. How did she respond?

• Students describe a scale model of the solar system built on the premise that the earth is represented by a ping-pong ball.

• Students make estimates of the number of times various events happen in an average lifetime, discuss their strategies for estimation, and then check their estimates against some reference. A good reference for this activity is In an Average Lifetime by Tom Heymann. Among other things, in an average lifetime, an American consumes 10,231 gallons of beverages, spends $1,331 on home-delivered food, and spends 911 hours brushing his or her teeth.

13. Expand the sense of magnitudes of different number types to include integers, rational numbers, and roots.

• Students play Locate the Point. A number line with end points -5 and 5 is suspended in the classroom, using a long string with tabs to indicate the positions of the integers between the two end numbers. Students are given cards with different types of numbers on them. (For example: -12/3, 1.1, 1.01, sqrt(2), pi, -2², (-2)², sqrt(3), sqrt(8), 1.999..., 2, the cube root of 8, etc.) They take turns and attach their card on the appropriate spot on the number line. Classmates decide whether the position is correct. If more than one expression is used for the same number, the cards with those numbers are attached by tape.

• Students use only the multiplication and division functions on their calculators to perform a series of successive approximations to find acceptable values for several roots: the square roots of 2, 3, 7, and 10, and the cube roots of 10 and 100.

• Pairs of students play Hi-Lo with decimals as a way to emphasize the density of the rational numbers. One student thinks of a number between 0 and 10 with up to 4 decimal places. The other student tries to guess the number, receiving feedback after each guess as to whether the guess was too high or too low. Written records of the guesses and the feedback are kept. The goal is to find the number using as few guesses as possible.

14. Understand and apply ratios, proportions, and percents in a variety of situations.

• Students take consumer price data from 10 and 25 years ago and figure out the percentage increase or decrease in the prices of various products over those periods of time. They discuss questions such as: What makes a price go up? What would make it go down?

• Students predict, and then determine, which body part ratios are fairly constant from person to person. Some interesting ones are height/arm span, wrist circumference/hand span, and waist/neck circumference.

• Students make a three-dimensional model of the classroom with different groups taking responsibility for modeling different objects in the class. First the desired size of the model is discussed and a scale factor agreed upon. Then each of the groups measures and applies that scaling factor to their objects, determines appropriate materials and means of construction, and builds the models.

• Students examine whether it is better to take a discount of 20% and then add a 6% sales tax or add the sales tax and then take a 20% discount. (The answer may surprise the students!)

• Students examine different statements involving proportions and discuss which ones make sense and which do not. For example: If one girl can mow the yard in 30 minutes, then two girls can mow the yard in 15 minutes. If one boy can walk to school in 20 minutes, then two boys can walk to school in 10 minutes.

• Students compare magazine subscription prices for 6, 9, and 12 months in order to decide which is the better buy.

• Students estimate what percent of plain M&M's are red, green, yellow, blue, brown, and orange. They test their guesses by counting the number of each color in a small bag and finding the percentages. They also discuss whether they improve their estimates by combining their data.

• Students simulate running a business using the computer program The Whatsit Corporation or Survival Math.

15. Develop and use order relations for integers and rational numbers.

• Students use concrete and pictorial models to develop order relations among fractions and integers. Using Cuisenaire Rods and varying the unit, students demonstrate that one fraction is larger than another. Similar arguments and conclusions are made on a number line for integers.

• Students' abilities to order rational numbers (both positive and negative) are assessed by asking them to identify points on a number line between, say, -3 and -5.

• Students are each given a rational number on a large card (-1.2, 4, 3/4, -2 1/4, -1, 3.14, 22/7, and so on). They then order themselves from least to greatest along the front or side of the classroom. They also respond to instructions like: Hold up your card if it is between -2.5 and +0.7.

16. Recognize and describe patterns in both finite and infinite number sequences involving whole numbers, rational numbers, and integers.

• Students formulate a description of the nth row of Pascal's triangle.

• Students investigate the Fibonacci sequence (1, 1, 2, 3, 5, 8, 13, 21, ....) to see how it is generated and then do library research to find theories about its startling occurrences in nature.

• Students explore the golden ratio discovered and used by the ancient Greeks. They find examples of golden rectangles (whose sides are in the golden ratio) in everyday objects (3 x 5 cards, bricks, cereal boxes), and in architecture (the Parthenon).

• Students discuss and predict the sum of this well-known series:
  

17. Develop and apply number theory concepts, such as primes, factors, and multiples, in real-world and mathematical problem situations.

• Students write a Logo or a BASIC computer program to find all the factors of any number that is provided as input. They can then use the same program to determine if any input number is prime.

• Students explore Goldbach's conjecture (a mathematical hunch which has never been proved nor disproved) which states that: Any even number greater than two can be written as the sum of two prime numbers. For example: 14 = 11 + 3, 24 = 11 + 13, and 56 = 3 + 53. Can you find one that cannot be written this way?

• Students develop rules of divisibility for all one-digit numbers and explain and apply these rules on a test.

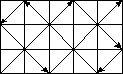
• Students investigate the path of a ball on a billiard table with sides of whole number length when the ball starts in a corner and always travels at a 45 degree angle. For example, a ball on the 3 x 5 table in the diagram starts in the lower left corner and takes the path shown, hitting the perimeter eight times (including the first and last corners) and going through all 15 squares, before ending at the top right corners. They make a table which records the length and width of the billiard table, the number of hits against the perimeter, and the number of squares passed through, for billiard tables of various sizes, and look for relationships. The number of hits against the perimeter of the table, including the first and last corners, is the sum of the width and the length of the billiard table divided by their greatest common factor.
  

18. Investigate the relationships among fractions, decimals, and percents, and use all of them appropriately.

• Given a circle graph of some interesting data, students estimate the size of each section of the graph as a fraction, a percent, and as a decimal. Students also create their own circle graphs.

• Students use two different-color interlocking paper circles (each has a cut along a radius so they fit together), each marked off in wedges that are one hundredth of the circle, to show fractions that have denominators of 10 or 100, decimals to hundredths, and whole number percents to 100%.

• Students explore patterns in particular families of decimal expansions, such as those for the fractions, 1/7, 2/7, 3/7, ... or 1/9, 2/9, 3/9, ... .

19. Identify, derive, and compare properties of numbers.

• Students work on this problem from the Curriculum and Evaluation Standards for School Mathematics (p. 93): Find five examples of numbers that have exactly three factors. Repeat for four factors, and then again for five factors. What can you say about the numbers in each of your lists?

• Students explore perfect numbers, those numbers that are equal to the sum of all of their factors including 1 but excluding themselves. Six is the first perfect number, where 6 = 1 + 2 + 3. Interestingly, the next one has 2 digits, the third has 3 digits and the fourth has 4 digits. The pattern breaks down there, though, since the fifth perfect number has 8 digits. Students who have worked on a computer program to find all of the factors of numbers (see Indicator 17 on the previous page) may want to revise their program to see how many perfect numbers they can find.

References

Heyman, Tom. On an Average Day. New York: Fawcett Columbine, 1989.

National Council of Teachers of Mathematics. Curriculum and Evaluation Standards for School Mathematics. Reston, VA, 1989.

Software

Logo. Many versions of Logo are commercially available.

Survival Math. Sunburst Communications.

The Whatsit Corporation. Sunburst Communications.

Video

Powers of Ten. Philip Morrison, Phylis Morrison, and the office of Charles and Roy Eames. New York: Scientific American Library, 1982.

On-Line Resources

http://dimacs.rutgers.edu/archive/nj_math_coalition/framework.html/

The Framework will be available at this site during Spring 1997. In time, we hope to post additional resources relating to this standard, such as grade-specific activities submitted by New Jersey teachers, and to provide a forum to discuss the Mathematics Standards.