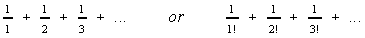STANDARD 6 - NUMBER SENSE
|
All students will develop number sense and an ability to represent
numbers in a variety of forms and use numbers in diverse
situations.
|
Standard 6 - Number Sense - Grades 9-12
Overview
High school students build upon their knowledge of rational numbers
as they increase their understanding of irrational numbers and
generalize number relationships through their work with algebra. The
key components of number sense, as identified in the K-12 Overview,
are an awareness of the uses of numbers in the world around us,
a good sense of approximation, estimation, and magnitude, the
concepts of numeration, and an understanding of the
equivalence of different representations and forms of
numbers.
In their work with numeration, ninth- through
twelfth-graders should view mathematics as a coherent body of
knowledge. They should see the integers, the rational numbers, and
the real numbers as logical and necessary extensions of the whole
number system. Only with these extensions can expressions like 3
minus 7, 4 divided by 3, and the ratio of the
circumference of a circle to its diameter have values. High
school students should understand how the integers, rational numbers,
irrational numbers, and real numbers are related to each other and
what properties are true for the numerical operations on these number
systems.
Students at these grade levels continue their learning about
equivalence, but with an increasing focus on approximation,
particularly for irrational numbers (see Standard 14 - Building
Blocks of Calculus). High school students should understand the
difference between an exact value of an irrational number, such as
sqrt(3), and its approximation (1.728). They should also be familiar
with the use of scientific notation as an equivalent form of decimal
number.
Estimation continues to be a regular part of mathematics
classes, both estimation of quantities and estimation of the results
of operations. Students should respond to questions that arise
naturally during the course of the class with answers that illustrate
confident and well-conceived use of estimation strategies and number
sense.
Technology also plays an important role in number sense at
these grade levels, particularly since calculators and computers use
approximations for some fraction-to-decimal conversions and for
irrational numbers. Calculators can be wonderful exploration tools
when examining numerical relationships, and computer software which
allows exploration of number relationships through conversion
utilities and graphs opens up even more possibilities.
The topics that should comprise the number sense focus of the ninth
through twelfth grade mathematics program are:
- the real number system
- exponents, roots, and scientific notation
- properties of number systems
Standard 6 - Number Sense - Grades 9-12
Indicators and Activities
The cumulative progress indicators for grade 12 appear below in
boldface type. Each indicator is followed by activities which
illustrate how it can be addressed in the classroom in grades 9, 10,
11, and 12.
Building upon knowledge and skills gained in the preceding grades,
experiences in grades 9-12 will be such that all students:
20. Extend their understanding of the number system to
include real numbers and an awareness of other number
systems.
- Students explore alternative number bases and note their
advantages and disadvantages. Binary and hexadecimal systems are of
primary interest because of their use in computer programming.
- Students read about and understand the historical
relationship between the square root of 2 and the Pythagoreans'
attempt to express the length of the diagonal of a square in terms of
its sides. They use geoboards to illustrate how the hypotenuse of a
right triangle can represent a length of sqrt(2), sqrt(3), or sqrt(5),
and so on.
- Students' understanding of real numbers is assessed by
asking them to locate rational and irrational numbers on a number
line.
- Students find two numbers between any two given real
numbers, such as .4222... and .424242... .
- Students can give examples of irrational numbers such as
3.010010001 ... or .4323323332 ... .
21. Develop conjectures and informal proofs of properties
of number systems and sets of numbers.
- Students discuss whether the transitive and reflexive
properties hold for different relationships, such as "is a friend
of", "is perpendicular to," or "is a factor
of."
- Students make up a number system using the symbols
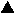 , ,
 , ,
 , ,
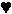 and and
 .
They develop algorithms for adding and multiplying within
their system and decide whether these operations are commutative and
associative. .
They develop algorithms for adding and multiplying within
their system and decide whether these operations are commutative and
associative.
- Students explore the properties of clock arithmetic or a
modular arithmetic system.
- Students examine properties involving addition of matrices,
scalar multiplication, and matrix multiplication. They demonstrate
that matrix multiplication is not commutative by providing a
counter-example.
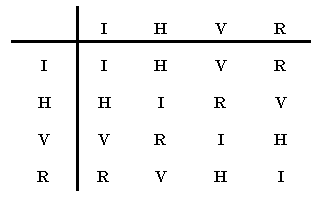
 Students investigate transformations of the rectangle ABCD:
reflection about its the horizontal line of symmetry (H), reflection
about its vertical line of symmetry (V), rotation by 180 degrees (R),
and rotation by 360 degrees (the identity, I). They construct an
operations table
(see below) which tells what happens if one of these
transformations is followed by another. Thus, for example, if you
reflect about the vertical line of symmetry (V) and then rotate by
180 degrees (R), the result is the same as reflecting about the
horizontal line of symmetry (H); this is indicated in the table by
placing H as the entry in the row for V and column for R representing
the conclusion that V followed by R is H. Students investigate
the properties of this operation "followed by."
Students investigate transformations of the rectangle ABCD:
reflection about its the horizontal line of symmetry (H), reflection
about its vertical line of symmetry (V), rotation by 180 degrees (R),
and rotation by 360 degrees (the identity, I). They construct an
operations table
(see below) which tells what happens if one of these
transformations is followed by another. Thus, for example, if you
reflect about the vertical line of symmetry (V) and then rotate by
180 degrees (R), the result is the same as reflecting about the
horizontal line of symmetry (H); this is indicated in the table by
placing H as the entry in the row for V and column for R representing
the conclusion that V followed by R is H. Students investigate
the properties of this operation "followed by."
22. Extend their
intuitive grasp of number relationships, uses, and interpretations and
develop an ability to work with rational and irrational
numbers.
- Students create computer spreadsheets to help assess
real-world and purely mathematical numerical situations and to ask
what if questions regarding complex data.
- Students use calculators and the formula for compound
interest to answer specific questions regarding the amount of money
that will be in a particular bank account after 1, 10, and 100
years.
- Students informally solve maximum/minimum problems with the
help of graphing calculators.
- Students use formulas for projectile motion to solve
problems regarding distance traveled, time in flight, maximum height,
and so on.
- Students compare different representations for pi,
including 3.14, 22/7, and the value given by their calculators. They
discuss the accuracy of each approximation, suggesting appropriate
circumstances for its use.
- Students work through the Ice Cones lesson
that is described in the First Four Standards of this
Framework. They use graphing calculations to determine the
maximum volume of a cone created from a 10 inch circle which is cut
along a radius.
23. Explore a variety of infinite sequences and
informally evaluate their limits.
- Students explore the value of .99 ... (or
4.999... or 3.2999...) as an infinite series (9/10 + 9/100 + ... ), and
conclude that its value is exactly 1 (or 5 or 3.3).
- Students analyze and discuss the sums of infinite series
such as the following:
- 1 - 1 + 1 - 1 + 1 - 1 + ...
- ( 1 - 1) + (1 - 1) + (1 - 1) + ...
- 1 + (- 1 + 1) + (-1 + 1) + (-1 + 1) + ...
- Students informally find the limits of real-world series
such as the total vertical distance traveled by a ball dropped from a
height of 10 meters which always bounces back to 3/4 of its original
height.
- Students investigate the sums of series such as:
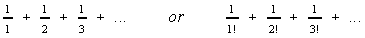
- Students estimate the area of a circle with radius of 10 by
informally judging the limiting value of the sequence produced by the
areas of inscribed regular polygons as their sides increase by 1; that
is, they calculate the area of an inscribed equilateral triangle, an
inscribed square, an inscribed pentagon, and so on.
- Students discuss pyramid schemes and create a
mathematical model to determine how many people would have to
participate in the scheme for everyone at the fifth level to be paid,
for everyone at the tenth level to be paid, and then for everyone who
participated at any level to be paid. For example, if each person
pays four others, then there are 4n people at the nth
level.
References
-
National Council of Teachers of Mathematics. Curriculum and
Evaluation Standards for School
Mathematics. Reston, VA, 1989.
On-Line Resources
-
http://dimacs.rutgers.edu/archive/nj_math_coalition/framework.html/
The Framework will be available at this site during Spring
1997. In time, we hope to post additional resources relating to this
standard, such as grade-specific activities submitted by New Jersey
teachers, and to provide a forum to discuss the Mathematics
Standards.
|
 ,
,
 ,
,
 ,
,
 and
and
 .
They develop algorithms for adding and multiplying within
their system and decide whether these operations are commutative and
associative.
.
They develop algorithms for adding and multiplying within
their system and decide whether these operations are commutative and
associative. Students investigate transformations of the rectangle ABCD:
reflection about its the horizontal line of symmetry (H), reflection
about its vertical line of symmetry (V), rotation by 180 degrees (R),
and rotation by 360 degrees (the identity, I). They construct an
operations table
(see below) which tells what happens if one of these
transformations is followed by another. Thus, for example, if you
reflect about the vertical line of symmetry (V) and then rotate by
180 degrees (R), the result is the same as reflecting about the
horizontal line of symmetry (H); this is indicated in the table by
placing H as the entry in the row for V and column for R representing
the conclusion that V followed by R is H. Students investigate
the properties of this operation "followed by."
Students investigate transformations of the rectangle ABCD:
reflection about its the horizontal line of symmetry (H), reflection
about its vertical line of symmetry (V), rotation by 180 degrees (R),
and rotation by 360 degrees (the identity, I). They construct an
operations table
(see below) which tells what happens if one of these
transformations is followed by another. Thus, for example, if you
reflect about the vertical line of symmetry (V) and then rotate by
180 degrees (R), the result is the same as reflecting about the
horizontal line of symmetry (H); this is indicated in the table by
placing H as the entry in the row for V and column for R representing
the conclusion that V followed by R is H. Students investigate
the properties of this operation "followed by."