In grades 5 and 6, students extend estimation to new types of numbers, including fractions and decimals. As indicated in the K-12 Overview, they continue to work on determining the reasonableness of results, using estimation strategies, and applying estimation to measurement, quantities, and computation.

In fifth and sixth grade, estimation and number sense are even more important skills than algorithmic paper-and-pencil computation with multi-digit whole numbers. Students should become masters at applying estimation strategies so that answers displayed on a calculator are instinctively compared to a reasonable range in which the correct answer lies.

The new estimation skills that are also important in fifth and sixth grade are skills in estimating the results of fraction and decimal computations. Even though the study of the concepts and arithmetic operations involving fractions and decimals begins before fifth grade, a great deal of time will be spent on them here. A sample unit on fractions for the sixth-grade level can be found in Chapter 17 of this Framework. As students develop an understanding of fractions and decimals and perform operations with them, estimation ought always to be present. Estimation of quantities in fraction or decimal terms and of the results of operations on those numbers is just as important for the mathematically literate adult as the same skills with whole numbers.

Children should understand that, sometimes, an estimate will be an accurate enough number to serve as an answer. At other times, an exact computation will need to be done, either mentally, with paper-and-pencil, or with a calculator to arrive at a more precise answer. Which procedure should be used is dependent on the setting and the problem. Even in cases where exact answers are to be calculated, however, students must understand that it is always a good idea to have an estimate in mind before the actual exact computation is done so that the computed answer can be checked against the estimated one.

The cumulative progress indicators for grade 8 appear below in boldface type. Each indicator is followed by activities which illustrate how it can be addressed in the classroom in grades 5 and 6.

Building upon knowledge and skill gained in the preceding grades, experiences in grades 5-6 will be such that all students:

5^*. Recognize when estimation is appropriate, and understand the usefulness of an estimate as distinct from an exact answer.

• Given pairs of real-life situations, students determine which is the one in which estimation is the best approach and which is the one needing an exact answer. For example, one such pair, might be: planning how long it would take to drive from Boston to New York and submitting a bill for mileage to your boss.

• Students collect data for a week on various situations when they and their families had to do some computation and describe when an exact answer was necessary (and why) and when an estimate was sufficient (and why).

• When doing routine problems, the students are always reminded to consider whether their answers make sense. For instance, in the following problem, an estimate makes much more sense than an exact computation. Molly Gilbert is the owner of a small apple orchard in South Jersey. She has 19 rows of trees with 12 trees in each row. Last year the average production per tree was 761.3 apples. At that rate, what can she expect the total yield to be this year? For this problem, an exact computation is certain to be wrong and will also be a number that is very hard to remember or use in further planning.

• Students look for examples of estimation language in their reading and/or in the newspapers.

• Students investigate what decisions at the school are based on estimates (e.g., quantity of food for lunch, ordering of textbooks or supplies, making up class schedules, organizing bus routes) by interviewing school employees and making written and/or oral reports.

6. Determine the reasonableness of an answer by estimating the result of operations.

• Students estimate the size of a crowd at a rock concert from a picture. They share all of their various strategies with the rest of the class.

• Students demonstrate their understanding by estimating whether or not they can buy a set of items with a given amount of money. For example: You have only $10. Explain how you can tell if you have enough money to buy: 4 cans of tuna @ 79 cents each, 2 heads of lettuce @ 89 cents each, and 2 lbs. of cheese @ $2.11 per lb.

• Students place decimal points in multi-digit numbers to make absurd statements more reasonable. Some typical statements could be Mr. Brown averages 2383 miles per gallon when he drives to work, My dog weighs 5876 pounds, or Big Burger charges $99 for a large order of french fries.

8. Develop, apply, and explain a variety of different estimation strategies in problem situations involving quantities and measurement.

• Students develop the concept of a billion by estimating its size relative to one hundred thousand, one million, and so on. For instance, they explore questions like If a calculator is programmed to repeatedly add 1 to the previous display and it takes about forty hours to reach a million, how long would it take to reach a billion? Students might relate this to the size of the national debt (now $5 trillion).

• Students estimate the area inside a closed curve in square centimeters and then check the estimate with centimeter graph or grid paper.

• After reading Shel Silverstein's poem "How Many, How Much" and Tom Parker's Rules of Thumb, students write their own rules of thumb (e.g., You should never have homework higher than one inch.).

• Students make estimates about things that happen in one day at school after reading about some of the data in In One Day, by Tom Parker. For example, they estimate: How much pizza is eaten? How much milk is drunk? How many students go home sick? or How many students forget something? They then interview people around the school or conduct a survey to check their estimates.

• Students develop strategies for estimating sums and differences of fractions as they work with them. For example, a fifth grade class is asked to determine which of the following computation problems have answers greater than 1 without actually performing the calculation; many students' strategies will hinge on comparisons of the given fractions to 1/2.
  

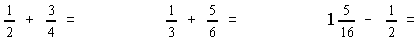
• Students make use of strategies like clustering and compatible numbers in estimating the results of computations. They recognize that a sum of numbers that are approximately the same, such as 37, 39, and 42, can be replaced in an estimate by the product 3 x 40 (clustering). They also know that other computations can be performed easily by changing the numbers to numbers that are closely related to each other, such as changing 468 divided by 9 to 450 divided by 9 (compatible numbers).

• Students work through the Mathematics at Work lesson that is described in the Introduction to this Framework. A parent discusses a problem which her company faces regularly: to determine how large an air conditioner is needed for a particular room. To solve this problem, the company has to estimate the size of the room.

9. Use equivalent representations of numbers such as fractions, decimals, and percents to facilitate estimation.

• Students use fractions, decimals, or mixed numbers interchangeably when one form of a number makes estimation easier than another form. For example, rather than estimating the product 3/5 x 4, students consider 0.6 x 4 which yields a much quicker estimate.

• In their beginning work with percent, students master the common fraction equivalents for familiar percentages and use fractions for estimation in appropriate situations. For example, an estimate of 65% of 63 can be easily obtained by considering 2/3 of 60.

10. Determine whether a given estimate is an overestimate or an underestimate.

• Students decide, as they discuss each new estimation strategy they learn, whether the strategy is likely to give an overestimate, an underestimate, or neither. For instance, using front-end digits will always give an underestimate; rounding everything up (as one might do to make sure she has enough money to pay for items selected in a grocery store) always gives an overestimate; and ordinary rounding may give either an overestimate or an underestimate.

• Students frequently use guess, check, and revise as a problem solving strategy. With this strategy, the answer to a problem is estimated, then calculations are made using the estimate to see whether this estimate meets the conditions of the problem, and the estimate is then revised upwards or downwards as a result. For example, students are asked to find two consecutive pages in a book the product of whose page numbers is 1260. An initial guess might be 30 and 31, the check might involve concluding that this product is a little over 900, and, as a result, they might revise their estimate upwards.

References

Parker, Tom. In One Day. Boston: Houghton Mifflin, 1984.

Parker, Tom. Rules of Thumb. Boston: Houghton Mifflin, 1983.

Silverstein, Shel. "How Many, How Much," in A Light in the Attic. New York: Harper and Row, 1981.

On-Line Resources

http://dimacs.rutgers.edu/archive/nj_math_coalition/framework.html/

The Framework will be available at this site during Spring 1997. In time, we hope to post additional resources relating to this standard, such as grade-specific activities submitted by New Jersey teachers, and to provide a forum to discuss the Mathematics Standards.