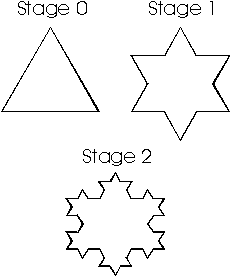STANDARD 7 - GEOMETRY AND SPATIAL SENSE
|
All students will develop spatial sense and an ability to use
geometric properties and relationships to solve problems in
mathematics and in everyday life.
|
Standard 7 - Geometry and Spatial Sense - Grades 7-8
Overview
Students can develop strong spatial sense from consistent
experiences in classroom activities that use a wide variety of
manipulatives and technology. The key components of spatial sense, as
identified in the K-12 Overview, are spatial relationships, properties
of geometric figures, geometric transformations, coordinate geometry,
geometry of measurement, geometric modeling, and reasoning.
Students in grades 7 and 8 learn geometry by: engaging in
activities and spatial experiences organized around physical models,
modeling, mapping, and measuring; discovering geometric relationships
by using mathematical procedures such as drawing, sorting,
classifying, transforming, and finding patterns; and solving geometric
problems.
Building explicit linkages among mathematical topics is especially
important with respect to geometry, since geometric concepts
contribute to students' understanding of other topics in
mathematics. For example, the number line provides a way of
representing whole numbers, fractions, decimals, integers, lengths,
and probability. Regions are used in developing understanding of
multiplication, fraction concepts, area, and percent. The coordinate
plane is used to relate geometry to algebra and functions. Similar
triangles are used in connection with ratio and proportion.
Students continue to develop their understanding of spatial
relationships by examining projections (viewing objects from
different perspectives), shadows, perspectives, and maps. They apply
the understanding developed in earlier grades to solve problems
involving congruence, similarity, and symmetry.
Students begin to explore the logical interrelationships among
previously-discovered properties of geometric figures at
these grade levels. They extend their work with two-dimensional
figures to include circles as well as special quadrilaterals. They
continue to work with various polygons, lines, planes, and
three-dimensional figures such as cubes, prisms, cylinders, cones,
pyramids, and spheres.
The study of geometric transformations continues as well at
these grade levels, becoming more closely linked to the study of
algebraic concepts and coordinate geometry in all four
quadrants. Students begin to represent transformations and/or their
results symbolically. They also continue to analyze the relationships
between figures and their transformations, considering congruence,
similarity, and symmetry.
The geometry of measurement is extended to circles,
cylinders, cones, and spheres in these grades. Students learn about
pi and use it in a variety of contexts. They explore different
ways to find perimeters, circumferences, areas, volumes, surface
areas, and angle measures. They also develop and apply the
Pythagorean Theorem. The emphasis is on understanding the processes
used and on recording the procedures in a formula; students should not
simply be given a formula and be expected to use it.
Students continue to use geometric modeling to represent
problem situations in different areas. Drawings of various types are
particularly useful to students in understanding the context of
problems. Number lines, coordinate planes, regions, and similar
triangles help students to visualize numerical situations. Especially
important are the patterns produced by change processes, including
growth and decay.
Students further develop their reasoning skills by making
conjectures as they explore relationships among various shapes and
polygons. For example, as students learn about the midpoints of line
segments, they can make guesses about the shapes produced by
connecting midpoints of consecutive sides of quadrilaterals. By
testing their hypotheses with drawings they make (by hand or using a
computer), the students come to actually see the possibilities that
can exist. The informal arguments that students develop at these grade
levels are important precursors to the more formal study of geometry
in high school.
The emphasis in grades 7 and 8 should be on investigating and using
geometric ideas and relationships, not on memorizing definitions and
formulas. A special feature of these grade levels is that students
are preparing to take the New Jersey Early Warning Test (EWT). Many
of the items in the Measurement and Geometry Cluster of the EWT will
ask students to use those geometric ideas and relationships to solve
problems - not simply to recall formulas. Indeed, the formulas
needed for the problems are given to them on the Reference Sheet that
accompanies the test. In their general classroom activity, as well as
in preparation for the EWT, students should use a variety of concrete
materials to model and analyze situations in two and three dimensions.
They should use drawings that they make, either by hand or with the
aid of a computer, to further examine geometric situations or to
record what they have done. Geometry approached in this way can be fun
and challenging for students.
Standard 7 - Geometry and Spatial Sense - Grades 7-8
Indicators and Activities
The cumulative progress indicators for grade 8 appear below in
boldface type. Each indicator is followed by activities which
illustrate how it can be addressed in the classroom in grades 7 and
8.
Building upon knowledge and skills gained in the preceding grades,
experiences in grades 7-8 will be such that all students:
11. Relate two-dimensional and three-dimensional
geometry using shadows, perspectives, projections, and
maps.
- Students build and draw solids made of cubes. They learn
to build solids from drawings and to make their own drawings of
solids. Among the drawings with which they should be familiar are the
two-dimensional flat view from top, front, and side; the
three-dimensional corner view; and the map view showing the base of
the building with the number of cubes in each stack. For example,
they can build the solid below; presented here are a three-dimensional
corner view, a flat view, and a map view.
- Students predict what the intersections of a plane with a
cylinder, cone, or sphere will be. Then they slice clay models to
verify their predictions.
- Students use cubes made of clear plexiglas and partially
filled with colored water to investigate cross sections of a plane
with a cube. They try to tilt the cube so that the surface of the
water forms various shapes, such as a square, a rectangle that is not
a square, a trapezoid, a hexagon, and others.
12. Understand and apply the concepts of symmetry,
similarity, and congruence.
- Students create three-dimensional symmetric designs using
cubes, cylinders, pyramids, cones, and spheres.
- Students build scale models of the classroom, using
similarity to help them determine the appropriate measures of the
models.
- Students use compasses and straight-edges to construct
congruent line segments and angles.
- Students work through the Sketching
Similarities lesson that is described in the First Four Standards
of this Framework. Students use a computer program and various
similar figures to discover that corresponding angles have equal
measures and corresponding sides have equal ratios.
13. Identify, describe, compare, and classify plane
and solid geometric figures.
- Students use toothpicks to construct as many different
types of triangles as possible, where each side of the triangles
consists of between one and five toothpicks. They record their
findings in a table, showing how many triangles are scalene,
isosceles, equilateral, right, and obtuse. They also indicate which
combinations of sides are impossible.
- Students sort collections of quadrilaterals according to
the number of lines of symmetry that each has.
- Students play clue games designed to help them distinguish
between necessary and sufficient conditions in describing a shape.
For example: If you want to challenge your friend to
identify a square by giving a set of clues, which minimum set of clues
would you select from the list below? Explain your
selection. Is it possible to select a different minimum
set of clues? Explain.
-
| - 4 right angles |
- all angles are 90 degrees |
| - 4 sides |
- all sides of equal length |
| - all angles congruent |
- opposite angles congruent |
| - opposite sides parallel |
- simple closed curve |
- Openended assessment items like those used on
the Early Warning Test can always be used to provoke discussion and
classroom activity. One of the sample items in the New Jersey
Department of Education's Mathematics Instructional Guide
(MG3) shows several figures and asks which of them can be put together
to form a square. The developmental and extension activities provided
there offer good suggestions for manipulative and transformation
tasks.
- Students work through the A Sure Thing !? lesson
that is described in the Introduction to this Framework. They
investigate the relationship among the measures of the interior angles
of a triangle by cutting out arbitrary triangles, tearing them into
three pieces so that each corner is intact, and fitting the corners
around a single point to make a straight angle.
- Students use diagrams to demonstrate the relationships
among properties. For example, they might draw a diagram to show the
logical relationship of ideas leading to the angle sum for a
quadrilateral, or, as below, to clarify the relationship among
different types of quadrilaterals.
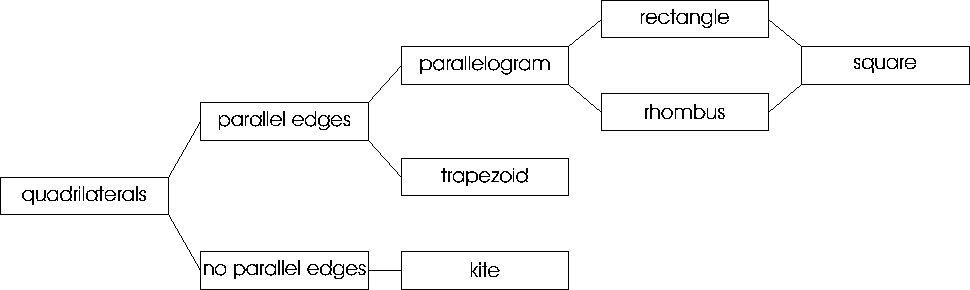
14. Understand the properties of lines and planes,
including parallel and perpendicular lines and planes, and
intersecting lines and planes and their angles of incidence.
- Students build a model of a cube, connect a midpoint of an
edge with a midpoint of another edge, and then connect two other
midpoints of edges to each other. They describe the relationships of
the segments they have constructed. They change one of the line
segments to another location and repeat the activity.
- Students identify congruent angles on a parallelogram grid,
using their results to develop conjectures about alternate interior
angles and corresponding angles of parallel lines and about opposite
angles of a parallelogram.
- Working in groups, students review geometric vocabulary by
sorting words written on index cards into groups and explaining their
reasons for setting up the groups in the way that they did.
15. Explore the relationships among geometric
transformations (translations, reflections, rotations, and
dilations), tessellations (tilings), and congruence and
similarity.
-
 Students use the "nibble" technique to create a shape
which will tessellate the plane, that is, copies of this shape will
fit together to cover a planar surface like a sheet of white oak-tag.
Start with a square, cut off a "nibble" along the top or
bottom edge of the square and
translate the nibble vertically to the opposite edge of the square;
the "nibble" will then be outside the boundary of the
original square. Take a "nibble" from the right or left
edge of the square and translate it horizontally to the opposite edge
of the square. Trace this shape repeatedly onto a sheet of white
oak-tag, by interlocking the pattern, and decorate the copies of the
shape. Attempt this process several times until a pleasing shape is
created.
Students use the "nibble" technique to create a shape
which will tessellate the plane, that is, copies of this shape will
fit together to cover a planar surface like a sheet of white oak-tag.
Start with a square, cut off a "nibble" along the top or
bottom edge of the square and
translate the nibble vertically to the opposite edge of the square;
the "nibble" will then be outside the boundary of the
original square. Take a "nibble" from the right or left
edge of the square and translate it horizontally to the opposite edge
of the square. Trace this shape repeatedly onto a sheet of white
oak-tag, by interlocking the pattern, and decorate the copies of the
shape. Attempt this process several times until a pleasing shape is
created.
- Students analyze the patterns found in Arabic designs such
as tiled floors and walls in Spain, identifying figures that represent
translations, reflections, and rotations. Then they generate their
own tile designs using basic geometric shapes. They can create their
own tile patterns using Tesselmania! software.
- Students apply transformations to figures drawn on
coordinate grids, record the coordinates of the original figure and
its image, and look for patterns. They express these patterns
verbally and symbolically. For example, flipping a point across the
x-axis changes the sign of the y-coordinate so that the point (x,y)
moves to (x,-y).
- Students practice doing geometric
transformations mentally by using the Geometric Golfer or
similar computer software. These programs present a series of puzzles
in which there is an object shape and a target shape.
The task is to use the fewest transformations possible to change the
object shape so that it is congruent to the target
shape. In the golf game, the object is a ball and the target is a
hole.
16. Develop, understand, and apply a variety of
strategies for determining perimeter, area, surface area, angle
measure, and volume.
- Students use a paper fastener to connect two models of rays
to form angles of different sizes. They estimate the correct
position, then measure their guess with a protractor to see how close
they were.
- Students are given a parallelogram-shaped piece of oak-tag
and asked to cut it apart and arrange the parts so that it is easy to
find its area. Their solutions are expressed verbally and
symbolically. This same process is repeated for a trapezoid.
- Students bring cans from home, arrange them by
estimated volume from smallest to largest, determine the actual
volumes by measuring and computing, and compare these results to their
estimates.
- Good conceptual assessment items designed to
measure students' understanding of area frequently ask the
students to find the area remaining in one figure after the area of
another figure has been removed. One sample item from the New Jersey
Department of Education's Mathematics Instructional Guide,
for example, asks students to find the area of a circular path that
surrounds a circular flower garden (MG1). Problems like this one are
not only good practice for tests like the Early Warning Test but can
also be used as informal assessments by teachers who listen carefully
to their students' discussions about them.
- Students work through the Rod Dogs lesson
that is described in the First Four Standards of this
Framework. Students determine the effects of increasing the
dimensions of an object on its surface area and volume.
17. Understand and apply the Pythagorean
Theorem.
- Students draw right triangles on graph paper with legs of
specified lengths and measure the lengths of their hypotenuses. They
record their results in a chart and look for patterns.
- Students create a small right triangle in the middle of a
ten-by-ten geoboard or on dot paper and then build squares on each
side of the triangle. They record the areas of the squares and look
for a relationship involving these areas.
- Students use tangram pieces to build squares on each side
of the middle-sized triangular tangram piece. They then describe the
relationship among the areas of the three squares.
18. Explore patterns produced by processes of
geometric change, relating iteration, approximation, and
fractals.
- Students use the reducing and/or enlarging feature on a
copier to explore repeated reductions/enlargements by the same factor
(iteration).
- Students learn about the natural shapes that are produced
by growth. They investigate how nature produces complex structures in
which basic shapes occur over and over. For example, spider webs,
honeycombs, and snowflakes grow larger in a systematic way
(iteration). Students measure the age of a tree by looking at its
rings; this illustrates approximation. Students produce geometric
designs that illustrate these principles, as well as fractals, where
miniature versions of the entire design are evident within a
smallportion of the design.
- Students view the slides which accompany the
activity book, Fractals for the Classroom, Vol. 1, and
determine why each picture might have been included in a book about
fractals.
- Students make a table showing the perimeter of a Koch
snowflake (a type of fractal) and its area at each stage. They
discuss the patterns in the table. This is an example where the
perimeter increases without bound but the area approaches a
limit.
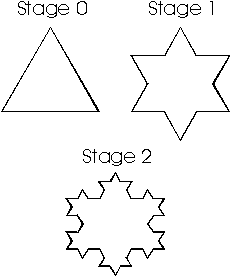
|
| Stage |
Perimeter |
Area |
| 0 |
3 |
1 |
| 1 |
4 |
4/3 |
| 2 |
16/3 |
40/27 |
| 3 |
|
|
| 4 |
|
|
|
19. Investigate, explore, and describe geometry in
nature and real-world applications, using models,
manipulatives, and appropriate technology.
- Some students read and prepare a report and
presentation to the class on String, Straightedge, and
Shadow: The Story of Geometry by Julia Diggins. Starting with a
chapter about the presence of geometry in nature, this story traces
the history of geometric discoveries from the invention of early
measuring instruments.
- Students model decay in a bacterial culture by cutting a
sheet of grid paper in half repeatedly and recording the area of each
rectangle in a table. They then graph the number of cuts versus the
area to see an example of exponential decay.
- Students investigate the golden ratio
((1 + sqrt(5))/2) and its application to architecture (such as the
Parthenon), designs of everyday objects such as index cards and
picture frames, and its occurrence in pinecones, pineapples, and
sunflower seeds.
- Students write about why manufacturers make specially
designed containers for packaging their products, indicating how the
idea of tessellations might be important in the designs.
- Students use a computer program such as The Geometry
PreSupposer to investigate the relationship between the lengths of
the sides and the measures of the angles in isosceles, scalene, and
equilateral triangles.
- Groups of students prepare slide shows using slides from
Geometry in Our World to illustrate the connections between
geometry, science, and art.
- Pairs of students build kites of different shapes,
explaining to the class why they chose a particular shape. Each
student predicts which kite will fly highest, writing the prediction
in his/her journal. The class flies all of the kites, recording the
heights of each by using a clinometer and similar triangles.
- Students watch the video Donald in Mathmagic
Land. Although getting a bit dated, thisvideo still thrills most
viewers as Donald Duck encounters many animated applications of
geometry. Students then form teams which focus on an aspect of the
video and do further research on that application.
- Students read and choose projects to make from
the book Origami, Japanese Paper Folding by Florence
Sakade or some other origami instruction books. The detailed
instructions usually given in such books are rich in mathematical
language and discussions among the students should provide a setting
for the use of much geometric terminology.
References
-
Diggins, Julia. String, Straightedge, and Shadow: The Story
of Geometry. New York: Viking Press, 1965.
Engelhardt, J., Ed. Geometry in Our World. Reston, VA:
National Council of Teachers of Mathematics, 1992.
New Jersey Department of Education. Mathematics Instructional
Guide. D. Varygiannes, Coord. Trenton: 1996.
Peitgen, Heinz-Otto, et al. Fractals for the Classroom:
Strategic Activities, Vol. 1. Reston, VA: National Council of
Teachers of Mathematics, and New York: Springer-Verlag, 1991.
Sakade, Florence. Origami, Japanese Paper Folding.
Rutland, VT: Charles E. Tuttle Co., 1957.
Software
-
Geometric Golfer. Minnesota Educational Computing
Consortium (MECC).
The Geometry PreSupposer. Sunburst Communications.
Tesselmania! Minnesota Educational Computing Consortium
(MECC).
Video
-
Donald in Mathmagic Land. Walt Disney Studios. Los
Angeles: The Walt Disney Company, 1959.
General references
-
Geddes, D. Curriculum and Evaluation Standards for School
Mathematics: Addenda Series:
Geometry in the Middle Grades. Reston, VA: National
Council of Teachers of Mathematics, 1992.
Owens, D. T., Ed. Research Ideas for the Classroom: Middle
Grades Mathematics. New York: MacMillan, 1993.
On-Line Resources
-
http://dimacs.rutgers.edu/archive/nj_math_coalition/framework.html/
The Framework will be available at this site during
Spring 1997. In time, we hope to post additional resources
relating to this standard, such as grade-specific activities submitted
by New Jersey teachers, and to provide a forum to discuss the
Mathematics Standards.
|



 Students use the "nibble" technique to create a shape
which will tessellate the plane, that is, copies of this shape will
fit together to cover a planar surface like a sheet of white oak-tag.
Start with a square, cut off a "nibble" along the top or
bottom edge of the square and
translate the nibble vertically to the opposite edge of the square;
the "nibble" will then be outside the boundary of the
original square. Take a "nibble" from the right or left
edge of the square and translate it horizontally to the opposite edge
of the square. Trace this shape repeatedly onto a sheet of white
oak-tag, by interlocking the pattern, and decorate the copies of the
shape. Attempt this process several times until a pleasing shape is
created.
Students use the "nibble" technique to create a shape
which will tessellate the plane, that is, copies of this shape will
fit together to cover a planar surface like a sheet of white oak-tag.
Start with a square, cut off a "nibble" along the top or
bottom edge of the square and
translate the nibble vertically to the opposite edge of the square;
the "nibble" will then be outside the boundary of the
original square. Take a "nibble" from the right or left
edge of the square and translate it horizontally to the opposite edge
of the square. Trace this shape repeatedly onto a sheet of white
oak-tag, by interlocking the pattern, and decorate the copies of the
shape. Attempt this process several times until a pleasing shape is
created.