STANDARD 13 - ALGEBRA
|
All students will develop an understanding of algebraic concepts
and processes and will use them to represent and analyze relationships
among variable quantities and to solve problems.
|
Standard 13 - Algebra - Grades 7-8
Overview
Students can develop a strong
understanding of algebraic concepts and processes from consistent
experiences in classroom activities where a variety of manipulatives
and technology are used. The key components of this understanding in
algebra, as identified in the K-12 Overview, are: patterns,
unknown quantities, properties, functions, modeling real-world
situations, evaluating expressions and solving equations
and inequalities.
Students in grades 7 and 8 continue to explore algebraic concepts
in an informal way. By using physical models, data, graphs, and other
mathematical representations, students learn to generalize number
patterns to model, represent, or describe observed physical
patterns, regularities, and problems. These informal explorations
help students gain confidence in their ability to abstract
relationships from contextual information and use a variety of
representations to describe those relationships. Manipulatives such
as algebra tiles provide opportunities for students with different
learning styles to understand algebraic concepts and manipulations.
Graphing calculators and computers enable students to see the
behaviors of functions and study concepts such as slope.
Students need to continue to see algebra as a tool which is useful
in describing mathematics and solving problems. The algebraic
experiences should develop from modeling situations where
students gather data to solve problems or explain phenomena. It is
important that all concepts are presented within a context, preferably
one meaningful to students, rather than through traditional symbolic
exercises. Once a concept is well-understood, the students can use
traditional problems to reinforce the algebraic manipulations
associated with the concept.
Many activities which are used in earlier grades should be
revisited as students become more sophisticated in their use of
algebra. At the same time, activities used in later grades can be
incorporated on an informal basis. For example, students in earlier
grades might have gathered the heights and armspans and attempted to
generalize the relationship between them in words. As students became
familiar with the rectangular coordinate system, they might have
generalized the relationship using a scatterplot and fitting a line to
the data. In seventh and eighth grade, students might be taught how
to find the medianmedian line to determine the line of best fit and
use that line to solve problems. In later grades, when students have
learned to find the slope of a line through two points symbolically,
they can determine the equation of the median-median line. (The
median-median line, available on many calculators which have
statistics capabilities, is found by dividing the data points on the
x-y plane into three equal sets, grouped by x-value, finding a single
point for each set whose coordinates are the medians of the respective
coordinates of the points in the set, connecting the first and third
points by a straight line, and shifting this line 1/3 of the way
toward the second point.)
Students should have numerous opportunities to develop an
understanding of the relationship between a function and its graph. A
limited number of functions should be plotted by hand, but students
should also use technology to graph functions. While linear
relationships should be the focus, inequalities and nonlinear
functions should be explored as well. Students should develop an
understanding of the relationship between solutions of equations and
graphs of functions. For example, the solution of the equation 3
x - 4 = 5 can be found by plotting y = 3x
- 4, tracing along the function until a y-value of 5is
found, and then determining the corresponding x-value. Students
should develop the ability to find solutions using the trace function
of graphing calculators and computer graphing programs and discuss how
it assists in solving equations. They should also have opportunities
to use spreadsheets as a method for representing and solving problems.
Students should be able to evaluate expressions using all
forms of real numbers when calculators are available. They should
have developed an understanding of the importance of the algebraic
order of operations and be able to correctly evaluate expressions
using it. It is imperative that students understand that they cannot
blindly accept answers produced on the calculator. They should
recognize that a standard fourfunction calculator does not use the
standard order of operations. They should recognize that even with a
scientific calculator, operations such as the division of two binomial
quantities requires the use of parentheses.
Students should refine their ability to solve simple linear
equations (i.e., ax+b=cx + d). Students may continue to
use informal, concrete, and graphic methods but should begin to link
these methods to more formal symbolic methods. As students have
opportunities to explore interesting problems, applications, and
situations, they need to be encouraged to reflect on their
explorations and summarize concepts, relationships, processes, and
facts that have emerged from their discussions. Developing a suitable
notation to describe these conclusions leads naturally to a more
formal, more symbolic view of algebra.
Standard 13 - Algebra - Grades 7-8
Indicators and Activities
The cumulative progress indicators for grade 8 appear below in
boldface type. Each indicator is followed by activities which
illustrate how it can be addressed in the classroom in grades 7 and
8.
Building upon knowledge and skills gained in the preceding grades,
experiences in grades 7-8 will be such that all students:
5. Understand and use variables, expressions,
equations, and inequalities.
6. Represent situations and number patterns with concrete
materials, tables, graphs, verbal rules, and standard algebraic
notation.
- The seventh grade is preparing for a skiing trip. The
interdisciplinary team has decided to integrate the planning for the
trip into all the courses. One of the items being discussed in math
class is the number of buses that will be required. Since the actual
number of people is not yet determined, the situation is best modeled
with variables and unknowns. Knowing that each bus holds 35 people,
students develop a table based on 5 or 6 different numbers of people
on the trip, in an attempt to find a pattern. Some students who
require more concrete operations to develop a sense of the pattern use
unit cubes and decimal rods to represent the situation with different
numbers of people. The group works together to develop a graph based
on their findings. The discussion begins with one person
suggestinggraphing the points (35,1), (70,2), and
(105,3) and then connecting them with a straight line. The
teacher does this (in a way that can be readily erased later) and asks
the class if there is any problem with this solution. More discussion
leads to understanding that the graph would be made up of discrete
points since there cannot be fractions of people and the graph would
not be a straight line but a series of steps 35 people long and going
up by 1 bus. Students are able to verbalize the rule but are curious
as to how it would be represented symbolically. The teacher shows
them the symbol for the step function, _x_.
- Students construct squares on each side of right triangles
on their geoboards, then find the area of each square. They record
their results in a table and look for a pattern, leading them to
"discover" the Pythagorean Theorem.
7. Use graphing techniques on a number line to
model both absolute value and arithmetic operations.
- Students use a graphic representation of the mapping
resulting from a multiplication by a negative number (such as 2 as
shown below) to explain why the order of the inequality reverses when
both sides are multiplied by that negative number.
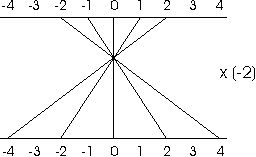
They explain that while the original sequence of inputs is
ascending {2,1,0,1,2}, the images are descending
{4,2,0,2,4}.
- Presented with an absolute
value equation such as | x - 5 | < 3, the students use the
idea that this means identifying all the points which are 3 units or
less from 5. They represent the solution set with dots at 2 and 8 and
a line connecting them.
- Students represent multiplication of integers on the
number line as repeated addition. They use the idea that 3
× 4 is 4 + 4 + 4, and, on the number line, it
would be represented by three segments, each four units in length,
placed end to end starting at zero. The students write in their
journals how they would picture 3 × (4).
After reviewing their responses and clarifying concerns, they discuss
that (3) × (4) would be the opposite
of 3 × (4).
8. Analyze tables and graphs to identify
properties and relationships.
9. Understand and use the rectangular coordinate
system.
- Students draw the quadrilateral ABCD, where the
coordinates of the vertices are A(3,2), B(4,7), C(2,3), and D(5,6).
They produce the figure that results from a size change of 1/2 and
then slide that quadrilateral left 3 units and down 5.
- Students understand that the point (5,3) is the
intersection of two lines which have the equations x=5 and
y=3. They can identify quickly lines with equations
such as x=5 and y=3. They can identify the half
planes and intersections of half planes identified by inequalities
such as x>5 and y< 3.
- Students study the relationship between perimeters and
areas of rectangles. Some students keep the perimeter constant and
study the changes in area while others keep the area constant and
study the changes in perimeter. Both groups plot their results as
graphs and look for patterns.
- Students use squares or grid paper to study the
relationship between the radius of a circle and its area (found by
counting squares on centimeter grid paper). They graph their data and
use it to predict the area of a circle of radius r.
- Students play Green Globs on the computer, entering
equations and trying to hit as many globs as possible with them.
10. Solve simple linear equations using
concrete, informal, and graphical methods, as well as
appropriate paper-and-pencil techniques.
- Presented with a picture of a balance scale showing
objects with unknown weights on both sides as well as known weights
(e.g., 3x + 12 = 7x + 4), students identify the standard
algebraic equation related to the picture, describe in words how it
would be solved using the concrete objects on the balance scale, and
record their actions symbolically.
- Students use algebra tiles to solve an equation like
3(2x + 5) = 21. They first place 21 units on the right and
three groups of two strips and five units on the left. They then note
that this is the same as saying 6 strips and 15 units balance 21 units
(6x + 15 = 21). Then they take 15 units off both sides, leaving
6 strips balanced with 6 units (6x = 6). They conclude that one
strip must equal one unit (x = 1).
- As part of a regular exam, students write a verbal
explanation describing the relationship between the function
y=2x+4, its graph, and the equation 2=2x+4.
11. Explore linear equations through the use
of calculators, computers, and other technology.
- The students perform an experiment to answer the question
about how long it would take a wave to go around Veterans'
Stadium. They gather data by timing waves done by a various
numbers of people, plot the data, and determine the line of best fit
using the medianmedian line method. They determine the number of
people that could sit around Veterans Stadium, but they discover that,
unlike the previous data sets, they cannot use the graph to answer the
question directly. The teacher explains that they need to determine
an equation for the line. She has the students investigate functions
of the form y=mx+b using a graphing calculator in order to
develop the idea that m represents the slope and b
represents the y-intercept.
- Using a motion detector connected to a computer or
graphing calculator, the teacher has students walk so that the
distance from the detector plotted against time is a straight
line. The teacher gives directions to students such as: Walk so
that the line has a positive slope, Walk so that the slope is
steeper than the last line, Walk so that the line has a slope of
0, or Walk so that the line has a negative slope.
12. Investigate inequalities and nonlinear
equations informally.
- Presented with the information that the Cape MayLewes
Ferry has space for 20 cars, and a bus takes up the space of 3 cars,
students are asked to draw a graph which represents how many cars and
how many buses can be taken across on one trip. Students use
variables to represent the unknowns (x for cars and y for
buses) and develop the inequality x+3y < 20 as a model for
the situation. Recognizing that the solutions have to be whole
numbers, they identify the points whose coefficients are non-negative
integers and in the first quadrant on or below the line.
- Students use a motion detector, connected to a calculator
or computer, to record the motion of a ping-pong ball tossed by a
small catapult. The motion detector is on the floor below the
trajectory of the ball. Students note that the graph of distance
against time is not linear. They experiment with different initial
velocities and different release points to see how these affect the
graph.
13. Draw freehand sketches of, and interpret,
graphs which model real phenomena.
- Students are asked to draw a sketch of the graph which
would describe a person's distance off the ground during a ride
on a ferris wheel which had a radius of 60 feet. Some students just
draw a curve that looks similar to a sine curve. Others put more
detail into their drawing showing the step function behavior which
occurs as people get on and get off and that there are limited
revolutions permitted.
- Presented with a graph showing the population of frogs in
a local marsh over the past ten years, students generate hypotheses
for why the curve has the shape it does. They check their hypotheses
by talking with a local biologist who has studied the marsh over this
time period.
References
-
New Jersey State Department of Education. Mathematics
Instructional Guide. D. Varygiannes, Coord. Trenton:
1996.
Software
-
Green Globs and Graphing Equations.
Sunburst Communications.
On-Line Resources
-
http://dimacs.rutgers.edu/archive/nj_math_coalition/framework.html/
The Framework will be available at this site during Spring
1997. In time, we hope to post additional resources relating to this
standard, such as grade-specific activities submitted by New Jersey
teachers, and to provide a forum to discuss the Mathematics
Standards.
|